43) Bonds
Ten Plus Bonds: Place Value Partitioning
Mathematics
Develop fluency:
- Writing numbers up to 29, that have been partitioned using place value.
- Representing numbers up to 29, with Bond Blocks, partitioned using place value.
Language
- addition as “and”
- equals as “is”
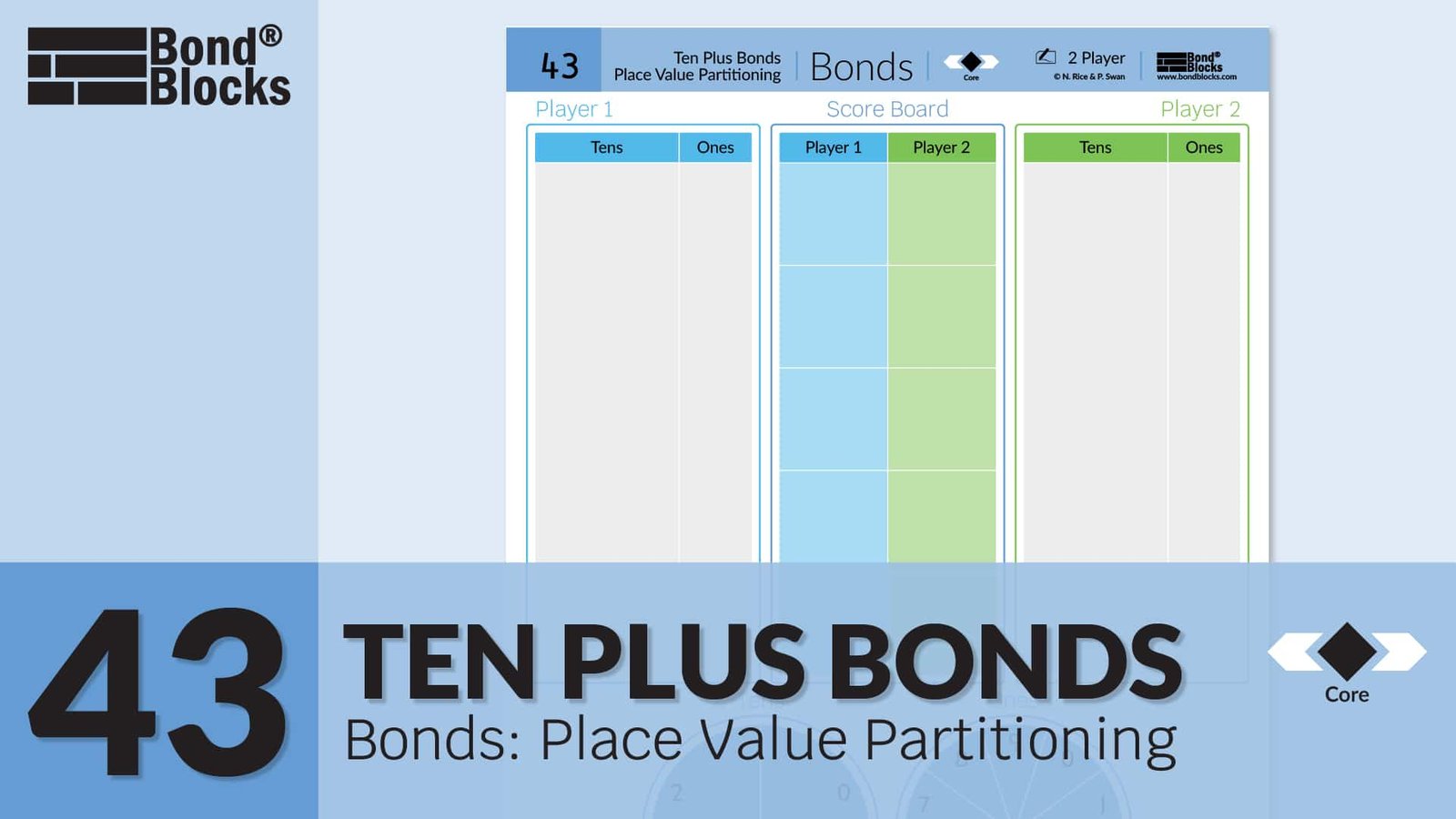
- A number is made up of digits in the same way a word is made up of letters. The number 23 is made up of the digits 2 and 3.
- Place value: The place of a digit in a number determines the digit’s value (worth).
- In the number 23 the digit 2 is in the tens place, so is worth 2 tens, 20.
- The digit 3 is in the ones place, so is worth 3 ones, 3.
- greater than. For example, 23 is greater than 18.
- less than. For example, 18 is less than 23.
The correct mathematical language when comparing numbers is “greater than” and “less than”.
- For example, “Five is greater than three.”
- These terms are used in nationwide assessments from the earliest years of schooling.
- These are the appropriate terms when referring to Bond Blocks. Bond Blocks are based on length. Length is a form of continuous measure, as is a number line.
- The terms “greater” and “less” are used for continuous measure.
The terms “bigger”, “larger” and “smaller”, in mathematics, refer to size. For example, area.
- When teaching we start with the learner’s understanding and build on this. Young students learn the words “bigger” and “smaller” in the context of their world. For example, “I am bigger than my baby brother”. Next they apply these words to numbers. For example, “Five is bigger than three”. Teachers can build on the student’s current understanding to help them progress mathematically by affirming and rephrasing, “Yes, 5 is greater than 3.”
- Whilst the 5 Bond Block is in fact “bigger” than the 3 Bond Block, the aim is to move students from the representational block to the abstract number, saying “Five (abstract number) is greater than three”.
The terms “fewer” and “more” refer to discrete, countable objects.
- For example, counters.
- These terms are not appropriate for Bond Blocks.


Differentiation
A little easier
Concrete-Representational-Abstract: place value arrow cards
Click to download Place Value Arrow Cards. Use these in conjunction with Bond Blocks to help students make connections between the manipulatives and written value of each digit.

Explicit Connections: representing numbers
Communicating with numbers involves making connections between a range of skills. These include:
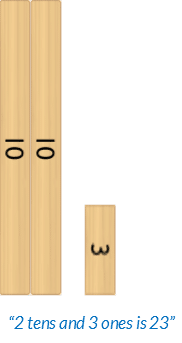
- Making a number. For example, with Bond Blocks
- Reading a number. For example, asking a student to point to twenty-one on a number board.
- Saying a number. For example, “twenty one”.
- Writing a number. For example, 21.
Whilst these skills are related, they are also separate. Just because students can do one skill correctly does not mean they can do the others.
Once students make correct mathematical connections between the said name, written number and physical quantity then check they can write the number using numerals. Writing the number with numerals correctly is a separate skill.
- Students with directional difficulties may incorrectly write 40 as
- Students with a partially developed concept of place value might write numbers as they hear them. For example, 21 written incorrectly as 201 because the student has heard ‘20’ and ‘1’. Use place value arrow cards in conjunction with Bond Blocks to teach correct writing using place value.
Students who experience ongoing difficulties writing numbers can be assisted by using place value arrow cards, number stamps, number tiles or electronic key pads as visual alternatives to handwriting. Replacing handwriting for these students enables them to focus on the mathematics. Correct digit formation can be focused on in a separate lesson or as instructed by the student’s occupational therapist. Adjustments for writing might be helpful for some students with directional difficulties, fine motor difficulties or those who learn best focusing on one mode of output at a time. This may include some students with dyslexia, dyscalculia, dysgraphia, dyspraxia, ADHD and ASD.
A little harder
Calculate: difference
Play as per Bonds: Place Value Partitioning except the player scores how many more they need to build 30. That is, the difference between the number made and 30.
Instructions
Player 1:
- Flick the tens spinner, then the ones spinner.
- Collect the matching Bond Blocks.
- Place the blocks in the correct columns.
- Record how many more blocks are needed to make 30.
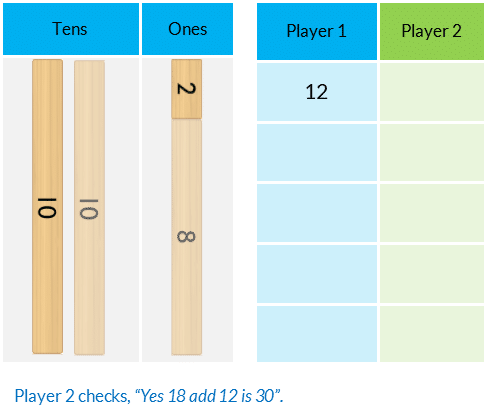
For example, Player One spun 1 ten and 8 ones, so scored 12.
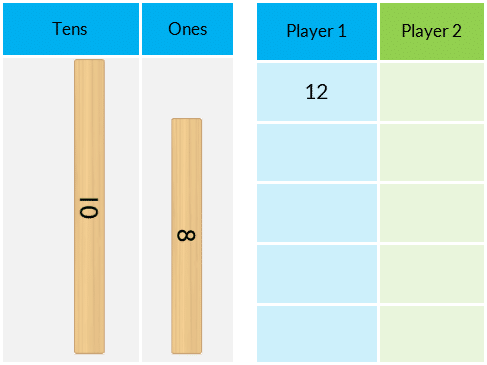
Player 2 uses Bond Blocks to check Player One’s score.
The player with the greatest score after each round wins.
The winner circles their number to keep track of the win.
The overall winner is the player with the most number of rounds won.
Calculate: difference
Play as per Bonds: Place Value Partitioning except the player scores how many more they need to build 30. That is, the difference between the number made and 30.
Instructions
Player 1:
- Flick the tens spinner, then the ones spinner.
- Collect the matching Bond Blocks.
- Place the blocks in the correct columns.
- Record how many more blocks are needed to make 30.
For example, Player One spun 1 ten and 8 ones, so scored 12.

Player 2 uses Bond Blocks to check Player One’s score.

The player with the greatest score after each round wins.
The winner circles their number to keep track of the win.
The overall winner is the player with the most number of rounds won.
Progression
In the next activity students apply ten plus bonds to addition and subtraction. Go to
Activity 44
Ten Plus Bonds: Addition and Subtraction, Ten and One

